Vlad Mărgărint

Research Interests
  • Mathematical Physics: Schramm-Loewner evolutions, Statistical Mechanics;
  • Random Matrix Theory; Rough Path Theory.
  • Study of the properties of Random Riemann Zeta functions, Random Dirichlet L-functions, classes of Random L-functions,- probabilistic analogues of (generalized) Riemann Hypothesis type problems.
  • (Biased) Random walks on random graphs and their scaling limits;
News: 1)In January 2026, following the generous invitation of the UNAM Mexico, I will deliver a one-week mini-course on 'SLE Theory, Random Matrix Theory and a bridge between them' based on our research. To the best of my knowledge, this is the first mini-course touching on both areas aiming at describing a link between them. If you are interested, feel free to contact me.
2)In November 2025, following the generous invitation of the City University of Hong Kong, I will give a presentation on this recent work in Asia.
3)Recently gave talks at Duke University, IHES Paris, KTH Stockholm, Aalto University Finland, Rome La Sapienza University, University of Iasi, University of Bucharest, Budapest (Random Matrix Theory event) and Poland (SPA 2025). See [IHES Presentation] and [Duke University presentation] 4)For the [REU 2024] program I was joined by a student from Georgia Tech and one from Columbia University in the city of New York. 5) Our paper (12) that is described in the Research Snapshot 2) is featured in RMTA!
Research Overview:
My research explores several independent directions that are part of broad research programs at the intersection of Probability, Analysis, and Mathematical Physics. These include the rigorous analysis of Statistical Mechanics models; the study of stability, approximations, and pathwise behavior of Schramm–Loewner Evolutions (SLE) via Rough Path techniques; Random walks on Random Graphs, Random Matrix Theory (RMT) and its connections with SLE; as well as aspects of Probabilistic Number Theory. While each direction addresses distinct problems, together they reflect a broader focus on developing analytical and probabilistic methods to understand complex systems, scaling limits, probabilistic models of primes, and connections between seemingly distinct areas such as SLE and RMT. The sections following the Research Snapshot highlight these research programs and summarize recent results.
Research Snapshot:
1)[Update: 6th of October 2024] Modern Tools in the context of the Riemann Hypothesis type problems and study of the structure of the primes

A recent research program that I am exploring is centered on creating tools for studying Random Riemann Zeta functions, Random Dirichlet L-functions, classes of random L-functions, and their properties.

Potential probabilistic analogues of the Riemann hypothesis type problems in this context involves the study of existence of the set of zeros/number of zeros in a region of the Random Riemann Zeta functions for a given model of pseudo-primes. In contrast, one can study the zero-free regions of the Random Riemann Zeta functions for various models of pseudo-primes, and the interactions of their complements with the famous Re s=1/2 line. Similarly, for the random Dirichlet L- functions and multiplicative functions.

Given that these studies are based on probabilistic models of primes, this gives a link also towards the use of modern tools of Machine Learning in the study of these problems. As we are experiencing significant growth in these areas, we expect that these tools will help us in this study. In addition, the accuracy of these psuedo-primes models can be tested against the predictions on the zero-free regions of the Riemann Zeta function or other known estimates for the Riemann Zeta function. Similarly, for Dirichlet L- functions and multiplicative functions.
For more details, see paper (16).

2) Random Matrices and Schramm-Loewner Evolutions

This wide research program based on the toolbox below, explores one field with fundamental techniques from another, such as bringing in Loewner theory the nicer analytical object that is the resolvent, as an alternative to the analysis of the singular drivers dynamics, .etc. This extends my other research program on pathwise study, approximations and stability in SLE theory described later on this page.

My research journey started with two projects in Random Matrix Theory (RMT). RMT has applications ranging from Physics, Biology to Neural Networks and it is an area of active research!
Later, I moved to a different area and started studying Schramm-Loewner Evolutions Theory (SLE), another great and active area of research, which I studied with techniques from Rough Path Theory, with Stochastic Analysis tools, and recently (see paper (8) below) with deterministic tools.

Although both SLE and RMT have been thriving areas of Probability Theory, these two fields have developed relatively independently.

We recently introduced a toolbox that connects the two areas and provides many potential new research directions and a platform for using techniques of one field to the other. The toolbox consists of seeing the right-hand side of the multiple SLE dynamics with Dyson Brownian motion driver as a normalized trace of a resolvent of a certain Random Matrix. This goes first by seeing the right-hand side of the multiple Loewner equation as a Stieltjes transform using the empirical measure on the drivers and then using the relation between this transform and the normalized trace of the resolvent of a Random Matrix. This normalized trace it is a very well-studied object in modern RMT, for example, in the proofs of Universality, and this gives us access to very good estimates. In addition, the toolbox beween RMT and SLE works also for fixed finite N number of cuvres. Given the interest for the fixed finite N number of curves model, we aim to explore technqiues from Non-Asymptotic RMT. In addition, for fixed N, identifying the matrix dynamics and studying its resolvent as the right-hand side provides an alternative to the analysis of the singular dynamics of the drivers, as the resolvent is a nicer analytical object. For more details see paper (12). See paper (5) also.

One of the advantages of this is that the hard critical parameters κ=4 and κ=8 in the SLE theory correspond to the β=2 and β=1 which are some of the nice well-studied cases in Random Matrix Theory.

3) Statistical Mechanics, Long-Range Models :

In a different direction, another major research program I explore aims at creating techniques to study the challenging long-range, general spins models and their scaling limits.

For more details, see papers (13) and (14). In a different direction, see also the recent work in paper (15).
Background

Tenure-Track Assistant Professor at the University of North Carolina at Charlotte (2023-)

Visiting Assistant Professor (via Burnett Meyer Fellowship) at the University of Colorado at Boulder (2022-2023)

Postdoctoral Fellow at NYU Shanghai (2019-2022)

DPhil (PhD) student in Mathematics, University of Oxford (2015-2019), under the supervision of Prof. Dmitry Belyaev and Prof. Terry Lyons.

MSc in Mathematics, ETH Zürich (between 2013-2015), under the supervision of Prof. Antti Knowles.

BSc in Mathematics, University of Bucharest (between 2010-2013), under the supervision of Prof. Victor Vuletescu.

Contact:
vmargari@charlotte.edu.
 Office 350E, Fretwell Building, University of North Carolina Charlotte, USA.

Here is my CV
The American Mathematical Society kindly accepted my proposal on an AMS Special Session on Schramm-Loewner Evolutions Theory and Random Matrix Theory, during the AMS 2026 Spring Eastern Sectional Meeting. See you in Boston in 2026! Feel free to contact me if you are interested!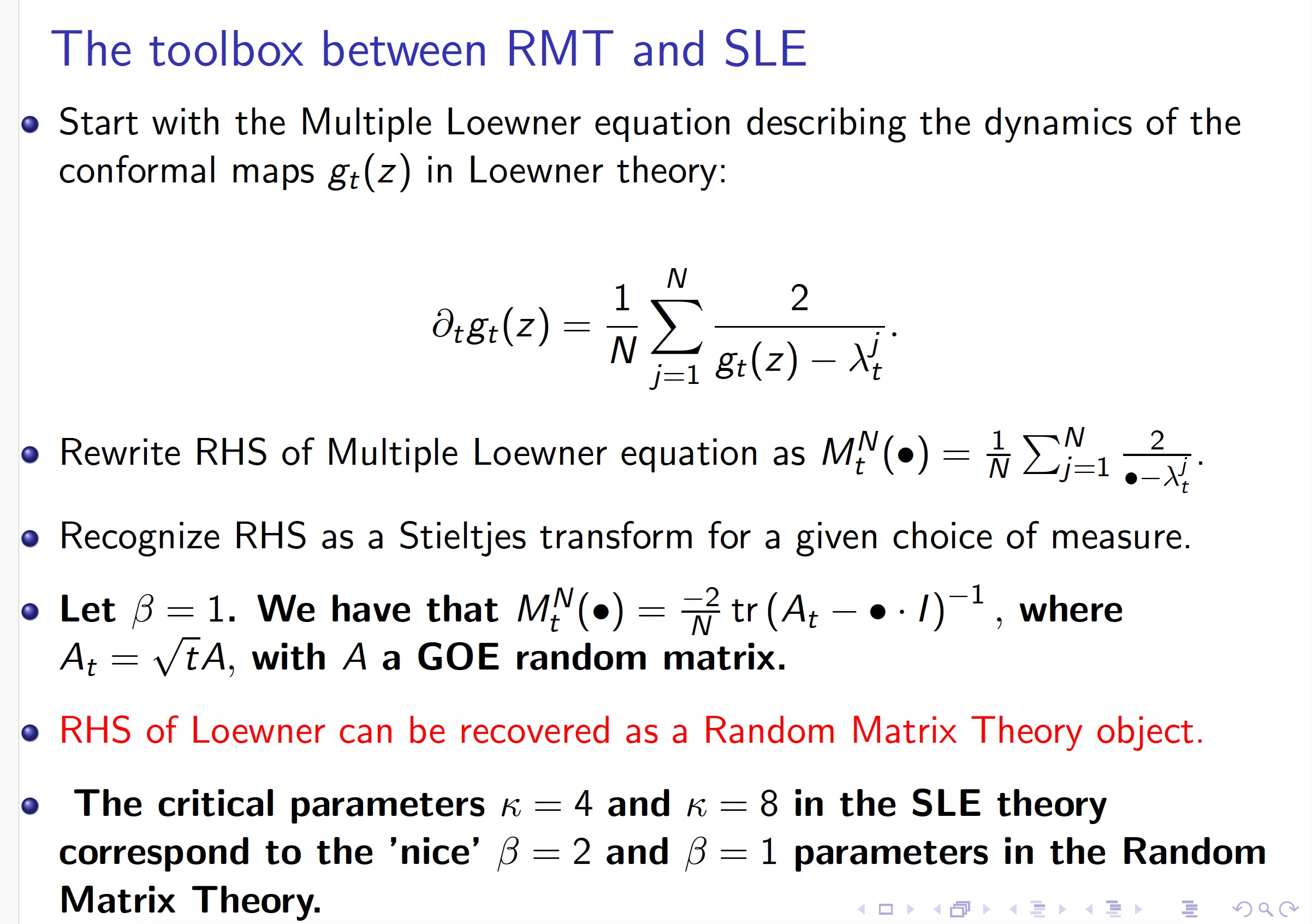

SLE and RMT illustration
Papers and preprints

    Work on Brownian motion on the Continuum Random Tree (Aldous' Conjecture stated in 1991- the year I was born)

  1. [New!-October 2024, 55 pages] On the cover time of Brownian motion on the Brownian continuum random tree [arXiv]-with G. Andriopoulos, David A. Crodyon, and Laurent Menard.

    2. Work in Probabilistic Number Theory -as part of the extended research program of developing tools and studying Random Riemann Zeta functions, their properties and zeros.

  2. On the analytic extension of Random Riemann Zeta Functions for some probabilistic models of the primes[arXiv]-with Stanislav Molchanov.

    Work in the mathematical analysis of Statistical Mechanics models -as part of the extensive research program to create tools for studying challenging long-range, general spins models and their scaling limits

  3. Scaling Limits of Disorder Relevant Non-Binary Spin Systems[arXiv]-with Liuyan Li and Rongfeng Sun (to appear in Stochastics and Partial Differential Equations: Analysis and Computations).
  4. Local Central Limit Theorem for unbounded long-range potentials[arXiv]-with Eric O. Endo, Roberto Fernandez and Nguyen Tong Xuan
  5. Local Central Limit Theorem for Two-Body Potentials at Sufficiently High Temperatures [Link]-with Eric O. Endo. (appeared in the Journal of Statistical Physics).

    6. Work at the interface between Schramm-Loewner Evolutions and Random Matrix Theory -as part of the wide research program aimed at studying one field with fundamental techniques from another such as bringing techniques corresponding to the nicer analytical object that is the resolvent, as an alternative to the analysis of the singular SLE drivers dynamics, .etc.

  6. Rate of Convergence in Multiple SLE using Random Matrix Theory [Link]-with A. Campbell and K. Luh (appeared in Random Matrices: Theory and Applications).

    7. Work on deterministic Loewner Theory, Schramm-Loewner Evolutions (and its connections with Rough Path Theory)-as part of the extensive research program to develop a pathiwse approach to SLE theory, and study approximations and stability in parameters of objects in SLE theory described by singular equations as analogies with the classical stochastic dynamics theory

  7. Splitting algorithm and normed convergence for drawing the random Loewner curves [arXiv]- with Jiaming Chen.( to appear in Proceedings of the Royal Society A).
  8. On Loewner chains driven by semimartingales and complex Bessel-type SDEs [Link]-with A. Shekhar and Y. Yuan (appeared in the Annals of Applied Probability)
  9. Convergence to closed-form distribution for the backward SLE at some random times and the phase transition at κ=8 [Link] - with Terry Lyons and Sina Nejad.( appeared in Statistics and Probability Letters).
  10. Deterministic Loewner Theory: Drivers, hitting times, and weldings in Loewner’s equation [Link]-with T. Mesikepp (appeared in the Journal of the London Mathematical Society)
  11. A Gaussian free field approach to the natural parametrisation of SLE4[Link]-with L. Schoug (appeared in Electronic Communications in Probability)
  12. Law of the SLE tip [Link]- with Oleg Butkovsky and Yizheng Yuan.( appeared in Electronic Journal of Probability).
  13. Perturbations of Simultaneously Growing Multiple Schramm-Loewner Evolutions [Link]-with Jiaming Chen.( appeared in Stochastic Processes and their Applications).
  14. Continuity of Zero-Hitting Times of Bessel Processes and Welding Homeomorphisms of SLE [Link] - with Dmitry Beliaev and Atul Shekhar. (appeared in ALEA- Latin American Journal of Probability and Mathematical Statistics).
  15. Continuity in κ in SLE theory using a constructive method and Rough Path Theory - with Dmitry Beliaev and Terry Lyons [Link] (appeared in Annales de l’Institut Henri Poincaré).
  16. An asymptotic radius of convergence for the Loewner equation and simulation of SLE traces via splitting [Link] - with James Foster and Terry Lyons. (appeared in the Journal of Statistical Physics)

    17. Work in Random Matrix Theory

  17. Convergence in High Probability of the Quantum Diffusion in a Random Band Matrix Model. appeared in Journal of Statistical Physics [Link] (under the supervision of Antti Knowles).
Videos, slides and posters
  • [New] Talk at the IHES, Paris 2025 [video]
  • [New] Talk at Duke University Probability Seminar 2025 [video]
  • Talk at the '10th World Congress in Probability and Statistics' 2021 [video]
  • Talk at the 'One World' Symposium [video]
  • Pathwise and probabilistic analysis in the context of SLE [PDF]
  • Using results on Bessel processes in the study of SLE [PDF]
  • Truncated Taylor approximation of Loewner dynamics [PDF]
  • Quantum Diffusion and Random Matrix Theory [PDF]
  • [P] Two results obtained in Random Matrix Theory [PDF]
  • Shapeletes and Compressive Sensing [PDF]
Expository Texts
  • An invitation to SLE Theory [PDF]
  • Introduction to Rough Paths Theory with applications [PDF]
  • Differentiable forms, integration and the Degree Theorem (Topology and Differential Geometry) [PDF]
  • Distribute Education Project (in Romanian) [PDF]
Teaching

UNC Charlotte:

Spring 2024: Probability Theory I (graduate course, part of Qual Exams) , 'STAT 2122 Introduction to Probability and Statistics' (Fall 2023).

CU Boulder:

Lector for 'Introduction to Probability and Statistics' (Fall 2022) and 'Linear Algebra for Non-Maths majors' (Fall 2022). Mini-course in 'Schramm-Loewner Evolutions' (Summer 2022).

NYU Shanghai:

Lector for Calculus (Summer course 2022) and for Mathematics for Economics II (Spring 2021) (mixed-mode) for NYU New York 'Go Local' students, Instructor for Calculus (mixed-mode) (Fall 2020), Honors Analysis I (online) (Spring 2020), Linear Algebra (online) (Spring 2020), Calculus (Fall 2019).

University of Oxford:

Tutor for: Numerical Analysis (Spring 2016); Stochastic Differential Equations (Winter 2017); Applied Probability (Winter 2017); Complex Analysis: Conformal maps and Geometry (Winter 2017); Continuous Martingales and Stochastic Calculus (Spring 2017); Statistical Mechanics (Winter 2017); Statistics and Data Analysis (Spring 2017, Spring 2018); Distribution Theory and Fourier Analysis (Winter 2018).

Teaching Assistant for: Approximations of functions (Winter 2015); Stochastic Analysis and PDEs (Spring 2016); Complex Analysis: Conformal maps and Geometry (Winter 2017).

ETH Zürich:

Methods of Mathematical Physics II (Spring 2014), Analysis I (Winter 2014), Analysis II (Spring 2013).

Past Travel 1: Isaac Newton Institute, Cambridge University, UK, August 2024. 24th Meeting of New Researchers in Statistics and Probability, Oregon, USA, August 2024. University of Chicago, IMSI Chicago- July 2024. SSP, Rice University, Texas, USA, March 2024. Alan Turing Institute London, University of Warwick, UK, March 2024, University of Chicago (November 2022), IAS Princeton and University of Pennsylvania (February 2023), University of Arizona -Seminar on Stochastic Processes Annual Conference of the IMS (March 2023), University of Utah (March 2023), The 24th Midrasha Mathematicae: Random Schrödinger Operators and Random Matrices, Israel Institute for Advanced Studies (May 2023), Jeju Island South Korea (workshop, June 2023), Paris (June 2023), 10th Congress of Romanian Mathematicians and First Balkan Mathematics Congress (Romania, July 2023). Past travel 2 : Kyoto University, Japan (2022), Indian Statistical Institute, New Delhi, India (2022), National University of Singapore (2022), University of Luxembourg (2020), Max Planck Institute Leipzig and TU Berlin (2020)
Links on my activity